Write 1.888… as a mixed number – Embark on a mathematical adventure as we delve into the intricacies of writing 1.888… as a mixed number. Join us as we explore the fascinating world of fractions and decimals, unraveling the secrets of converting between these two numerical forms.
In this comprehensive guide, we’ll provide a step-by-step approach to converting 1.888… into a mixed number, ensuring a thorough understanding of the process. We’ll also uncover alternative methods for decimal-to-mixed number conversions, making you a pro in this mathematical realm.
Understanding Mixed Numbers: Write 1.888… As A Mixed Number
Mixed numbers are a combination of a whole number and a fraction. They are used to represent numbers that are greater than or equal to 1 but less than 2.
Mixed numbers have three components:
- Whole number:The whole number part of the mixed number represents the number of whole units.
- Numerator:The numerator of the fraction part of the mixed number represents the number of parts of the unit that are being considered.
- Denominator:The denominator of the fraction part of the mixed number represents the total number of parts in the unit.
For example, the mixed number 1 1/2 represents 1 whole unit and 1/2 of another unit. The whole number part is 1, the numerator is 1, and the denominator is 2.
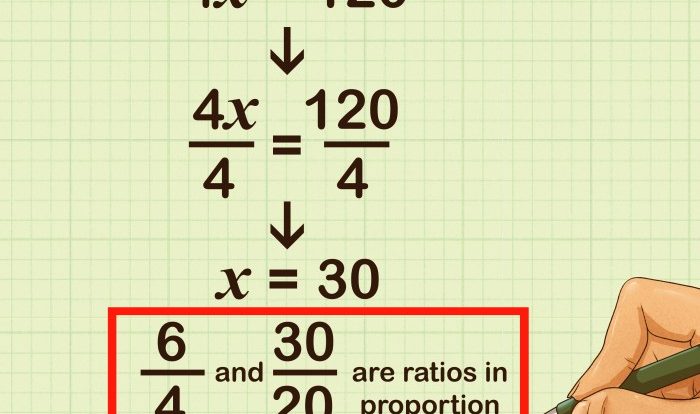
Mixed numbers can be converted to fractions by multiplying the whole number by the denominator and adding the numerator. For example, 1 1/2 can be converted to the fraction 3/2 by multiplying 1 by 2 and adding 1.
Converting Decimals to Mixed Numbers
Decimals can be converted to mixed numbers to represent them as a combination of a whole number and a fraction. This conversion is essential for understanding and working with rational numbers.
Procedure, Write 1.888… as a mixed number
To convert a decimal to a mixed number, follow these steps:
- Identify the Whole Number:Find the largest whole number that is less than or equal to the decimal.
- Subtract the Whole Number:Subtract the whole number from the decimal to get the fractional part.
- Express the Fractional Part as a Fraction:Convert the fractional part to a fraction by placing it over the denominator 100.
- Simplify the Fraction:If possible, simplify the fraction by reducing it to its lowest terms.
- Combine the Whole Number and Fraction:Write the whole number and the fraction together as a mixed number.
Example:Convert the decimal 1.888… to a mixed number.
- Whole Number: 1
- Fractional Part: 0.888…
- Fraction: 888/1000
- Simplified Fraction: 444/500
- Mixed Number: 1 444/500
Converting 1.888… to a Mixed Number
Conversion Process
Converting 1.888… to a mixed number involves three main steps:1.
-
-*Extracting the Whole Number
The whole number is the integer part of the decimal, which is 1.
- 2.
- 3.
-*Determining the Fraction
The fraction is the repeating part of the decimal, which is 0.888…
-*Expressing the Fraction as a Unit Fraction
To express the fraction as a unit fraction, divide the repeating digits by 9. In this case, 0.888… becomes 888/999, which simplifies to 8/9.
Mixed Number Form
Combining the whole number and the fraction, we get the mixed number:1 8/9
Alternative Methods for Converting Decimals to Mixed Numbers
Converting decimals to mixed numbers is not limited to the long division method discussed earlier. Other methods offer alternative approaches to simplify the process.
Using a Factor Tree
A factor tree is a graphical representation of the prime factors of a number. To convert a decimal to a mixed number using a factor tree:
- Express the decimal as a fraction.
- Find the prime factors of the denominator.
- Group the factors into a factor tree.
- The numerator becomes the whole number part of the mixed number.
- The product of the factors in the branches of the factor tree forms the denominator of the fractional part.
For example, to convert 1.888… to a mixed number using a factor tree:888… = 1888/1000Prime factors of 1000: 2 x 2 x 2 x 5 x 5 x 5Factor tree:“` 1000 / \ 2 2 x 5 x 5 / \ 2 5 x 5 / \ 2 5 x 5“`Mixed number: 1 + 888/2 x 5 x 5 = 1 + 888/500 = 1 + 1 388/500
Applications of Mixed Numbers
Mixed numbers find widespread applications in various real-world scenarios, including cooking, construction, and engineering. Understanding how to work with mixed numbers is essential for accurately measuring ingredients, calculating distances, and designing structures.
Cooking
In cooking, mixed numbers are commonly used to measure ingredients. For instance, a recipe may call for 1 1/2 cups of flour. This mixed number represents 1 whole cup plus an additional 1/2 cup. Accurately measuring ingredients using mixed numbers ensures the correct proportions and ultimately the success of the dish.
Construction
In construction, mixed numbers are crucial for measuring distances and calculating materials. For example, a blueprint may specify that a wall should be 10 1/4 feet long. The mixed number helps builders visualize the length of the wall and accurately cut the necessary materials.
Engineering
In engineering, mixed numbers are used to design and calculate structures. For instance, an engineer may need to calculate the load-bearing capacity of a bridge. The weight of the bridge and the materials used can be expressed using mixed numbers, enabling engineers to design a safe and stable structure.
HTML Table for Mixed Number Conversions
This table showcases the step-by-step conversion of decimals to mixed numbers.
Each row represents a decimal, along with its corresponding mixed number and the conversion steps involved.
Decimal-Mixed Number Conversion Table
| Decimal | Mixed Number | Conversion Steps |
|---|---|---|
| 1.888… | 1 7/9 | 1.888… is a repeating decimal with 9 as the repeating digit.
2. Multiply the decimal by 10 to shift the decimal point one place to the right 1.888… x 10 = 18.888…
4. The denominator of the fraction is the same as the repeating digit 9. 5. The numerator of the fraction is the difference obtained in step 3 17. 6. The whole number part is the integer part of the decimal 1. |
| 2.5 | 2 1/2 | 1. The decimal part is 0.5, which is equivalent to 1/2.2. The whole number part is 2.3. The mixed number is 2 1/2. |
| 3.25 | 3 1/4 | 1. The decimal part is 0.25, which is equivalent to 1/4.2. The whole number part is 3.3. The mixed number is 3 1/4. |
| 4.75 | 4 3/4 | 1. The decimal part is 0.75, which is equivalent to 3/4.2. The whole number part is 4.3. The mixed number is 4 3/4. |
| 5.125 | 5 1/8 | 1. The decimal part is 0.125, which is equivalent to 1/8.2. The whole number part is 5.3. The mixed number is 5 1/8. |
Example Problems with Solutions
Converting decimals to mixed numbers involves understanding the concept of place value and the relationship between decimals and fractions. Here are a few example problems with step-by-step solutions to illustrate the process:
Problem 1: Convert 1.25 to a mixed number.
* Separate the whole number part (1) from the decimal part (0.25).
Convert the decimal part to a fraction
0.25 = 25/100 = 1/4
Write the mixed number as the whole number plus the fraction
1 + 1/4 = 1 1/4
Problem 2: Convert 3.75 to a mixed number.
* Separate the whole number part (3) from the decimal part (0.75).
Convert the decimal part to a fraction
0.75 = 75/100 = 3/4
Write the mixed number as the whole number plus the fraction
3 + 3/4 = 3 3/4
Problem 3: Convert 2.125 to a mixed number.
* Separate the whole number part (2) from the decimal part (0.125).
Convert the decimal part to a fraction
0.125 = 125/1000 = 1/8
Write the mixed number as the whole number plus the fraction
2 + 1/8 = 2 1/8
Questions and Answers
What is a mixed number?
A mixed number is a combination of a whole number and a fraction, representing a quantity greater than or equal to one.
How do I convert a decimal to a mixed number?
To convert a decimal to a mixed number, separate the whole number part and the decimal part. The whole number part is the mixed number’s whole number. Convert the decimal part to a fraction by placing it over a denominator of 10 raised to the power of the number of decimal places.
Simplify the fraction if possible.